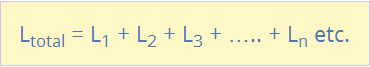INDUCTORS

INDUCTORS

An inductor, is a passive two-terminal electric component which resists changes in electric current passing through it. It consists of a conductor such as a wire, usually wound into a coil. When a current flows through it, energy is stored temporarily in a magnetic field in the coil. When the current flowing through an inductor changes, the time-varying magnetic field induces a voltage in the conductor, according to Faraday's law of electromagnetic induction,which opposes the change in current that created it.

An inductor consists of a coil of conducting wire.

If current is allowed to pass through an inductor, it is found that the voltage across the inductor is directly proportional to the time rate of change of the current. Using the passive sign convention,

where L is the constant of proportionality called the inductance.

Inductance is the property whereby an inductor exhibits opposition to the change of current flowing through it, measured in henrys(H).

The current-voltage relationship is obtained as,

The energy stored is, 

We should note the following important properties of inductor:

1. An inductor acts like a short circuit to DC.

2. The current through an inductor cannot change instantaneously.

3. The ideal inductor does not dissipate energy.

4. A practical, non-ideal inductor has a significant resistive component.

SERIES AND PARALLEL INDUCTORS:

Inductors in Series

Inductors can be connected together in either a series connection, a parallel connection or combinations of both series and parallel together, to produce more complex networks whose overall inductance is a combination of the individual inductors. However, there are certain rules for connecting inductors in series or parallel and these are based on the fact that no mutual inductance or magnetic coupling exists between the individual inductors.

                

 

The current, ( I ) that flows through the first inductor, L₁ has no other way to go but pass through the second inductor and the third and so on. Then, inductors in series have a Common Current flowing through them, for example:

                            I_L1 = I_L2 = I_L3 = I_AB …etc.

Inductors in series equation,

Then the total inductance of the series chain can be found by simply adding together the individual inductances of the inductors in series just like adding together resistors in series. However, the above equation only holds true when there is “NO” mutual inductance or magnetic coupling between two or more of the inductors.

Inductors in Parallel

Inductors are said to be connected together in “Parallel” when both of their terminals are respectively connected to each terminal of the other inductor or inductors. The voltage drop across all of the inductors in parallel will be the same. Then, Inductors in Parallel have a Common Voltage across them and in our example below the voltage across the inductors is given as:

                         V_L1 = V_L2 = V_L3 = V_AB …etc

In the following circuit the inductors L₁, L₂ and L₃ are all connected together in parallel between the two points A and B. 

 

Thus,

 Here, like the calculations for parallel resistors, the reciprocal ( 1/Ln ) value of the individual inductances are all added together instead of the inductances themselves. But again as with series connected inductances, the above equation only holds true when there is “NO” mutual inductance or magnetic coupling between two or more of the inductors. Where there is coupling between coils, the total inductance is also affected by the amount of coupling.

 

CAPACITORS

Capacitors

Capacitors are components that are used to store an electrical charge and are used in timer circuits. A capacitor may be used with a resistor to produce a timer. Sometimes capacitors are used to smooth a current in a circuit as they can prevent false triggering of other components such as relays. When power is supplied to a circuit that includes a capacitor - the capacitor charges up. When power is turned off the capacitor discharges its electrical charge slowly.
It is a passive element designed to store energy in its electric field. Besides resistors, capacitors are the most common electrical components. Capacitors are used extensively in electronics, communications, computers and power systems.
A capacitor consists of two conducting plates separated by an insulator.
                       
 When a voltage source v is connected to the capacitor, as shown in the figure below,

A capacitor with applied voltage.

 the source deposits a positive charge q on one plate and a negative charge - q on the other. The capacitor is said to store the electric charge. The amount of charge stored, represented by q, is directly proportional to the applied voltage v so that

where C, the constant of proportionality, is known as the capacitance of the capacitor.
Capacitance is the ration of the charge on one plate pf a capacitor to the voltage difference between the two plates, measured in farads.
To obtain the current-voltage relationship of the capacitor, we take the derivative of both sides.

 Differentiating both sides of equation.

The voltage-current relation of the capacitor can be obtained by integrating both sides of the equation above.

or

The energy stored in the capacitor is therefore,

 We should note the following important properties of a capacitor:
1. A capacitor is an open circuit to DC.
2. The voltage on a capacitor cannot change abruptly.
3. The ideal capacitor does not dissipate energy.
4. A real, non-ideal capacitor has a parallel-model leakage resistance.

SERIES AND PARALLEL CAPACITORS: 

When capacitors are connected in series, the total capacitance is less than any one of the series capacitors' individual capacitances. If two or more capacitors are connected in series, the overall effect is that of a single (equivalent) capacitor having the sum total of the plate spacings of the individual capacitors. As we've just seen, an increase in plate spacing, with all other factors unchanged, results in decreased capacitance.

                      


 Thus, the total capacitance is less than any one of the individual capacitors' capacitances. The formula for calculating the series total capacitance is the same form as for calculating parallel resistances:

                               


When capacitors are connected in parallel, the total capacitance is the sum of the individual capacitors' capacitances. If two or more capacitors are connected in parallel, the overall effect is that of a single equivalent capacitor having the sum total of the plate areas of the individual capacitors. As we've just seen, an increase in plate area, with all other factors unchanged, results in increased capacitance. 

                        
  
 Thus, the total capacitance is more than any one of the individual capacitors' capacitances. The formula for calculating the parallel total capacitance is the same form as for calculating series resistances:

                                   


 As you will no doubt notice, this is exactly opposite of the phenomenon exhibited by resistors. With resistors, series connections result in additive values while parallel connections result in diminished values. With capacitors, its the reverse: parallel connections result in additive values while series connections result in diminished values.

Maximum Power Transfer

Maximum Power Transfer

Suppose we have a voltage source or battery that's internal resistance is R_i and a load resistance R_L is connected across this battery . Maximum power transfer theorem determines the value of resistance R_L for which, the maximum power will be transferred from source to it. Actually the maximum power, drawn from the source, depends upon the value of the load resistance.

                  
Power delivered to the load resistance,


To find the maximum power, differentiate the above expression with respect to resistance R_L and equate it to zero. Thus,

A resistive load in a resistive network will abstract maximum power when the load resistance is equal to the resistance viewed by the load as it looks back to the network. Actually this is nothing but the resistance presented to the output terminals of the network. This is actually Thevenin equivalent resistance as we explained in Thevenin's theorem if we consider the whole network as a voltage source. Similarly, if we consider the network as current source, this resistance will be Norton equivalent resistance as we explained in Norton theorem.

 

 

TO UNDERSTAND MAXIMUM POWER TRANSFER: